- CAT Courses
- Free Video Resources & Tests
Video Resources
...
- CAT Past Papers
- Free Study Material
- Blogs
- Results
- Contact Us
Correct Answer
12
Correct Answer
1. 32 Units
Correct Answer
100
A square ABCD with side 8 has point E on AD, the value of BE+CE is definitely greater than:
Correct Answer
1. 8√5
Correct Answer
24
ABC is an equilateral triangle with O a point in it, with AO, BO and CO being 6,8,10. If triangle has an area of a√3 + b, find a+b.
Correct Answer
60
Correct Answer
24
Let P be the point of intersection of the lines 3x + 4y = 2a and 7x + 2y = 2018 and Q the point of intersection of the lines 3x + 4y = 2018 and 5x + 3y = 1. If the line through P and Q has slope 2, the value of a is:3 altitudes of triangle PQR have lengths 4,6 and 8 respectively, If P PQR has in-radius of length y, find 13y
Correct Answer
5. 3026
A triangle has its longest side as 38 cm. If one of the other two sides is 10 cm and the area of the triangle is 152 sq.cm, and the length of the third side is AVB,find the value of A+B .
Correct Answer
25Correct Answer
1:18
PQR is a right angled triangle where angle R is 90 degree. S is a point on PQ such that PS 29 and QS=1 and RS=13. Find area of triangle QRS.
Correct Answer
6
In the diagram, P,Q and R lie on a circle, the tangent at P and the secant QR intersect at T. The bisector of angle QPR meet at S so that QPS = RPS, If SQ=3 and PT = 4, Find length of RS
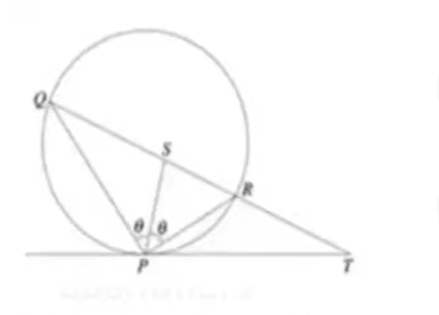
Correct Answer
12/7
Correct Answer
14√5
Correct Answer
120
If the area of a regular hexagon is equal to the area of an equilateral triangle of side 12 cm, then the length, in cm, of each side of the hexagon is:
Correct Answer
2. 2√6
Suppose the length of each side of a regular hexagon ABCDEF is 2 cm. It T is the mod point of CD, then the length of AT, in cm, is:
Correct Answer
1. √13
The sides AB and CD of a trapezium ABCD are parallel, with AB being the smaller side. P is the midpoint of CD and ABPD is a parallelogram. If the difference between the areas of the parallelogram ABPD and the triangle BPC is 10 sq cm, then the area, in sq cm, of the trapezium ABCD is:
Correct Answer
4. 30
If a rhombus has area 12 sq cm and side length 5 cm, then the length, in cm, of its longer diagonal is:
Correct Answer
2. √37 + √13
Let ABCD is a parallelogram. The lengths of the sides AD and the diagonal AC are 10 cm and 20 cm, respectively. If the angle ADC is 30 degree, then the area of the parallelogram, in sq. cm, is
Correct Answer
2. 25 (√3 + √15)
A park is shaped like a rhombus and has area 96 sq m. If 40 m of fencing is needed to enclose the park, the cost, in INR, of laying electric wires along its two diagonals, at the rate of ₹125 per m, is:
Correct Answer
3500
Correct Answer
4. 80
In ΔABC, ∠B = 900. PQRS is a square of side 5 units and WXYZ is a square of side 10 units. MS and SY are the length and breadth respectively of rectangle MNSY. What is the length of MS?
Correct Answer
2. 15 cm
The vertices of a triangle are (0, 0), and (4, 0) and (3, 9). The area of the circle passing through these three points is:
Correct Answer
3. 205π /9
In triangle ABC, M is the midpoint of the side AB. N is point in the interior of aking triangle ABC, such that CN is the bisector of angle C and CN is perpendicular to NB. What is the area of triangle BMN and AMN if CN=6, BC= 10cm and AC = 15cm?
Correct Answer
6
Correct Answer
20
Correct Answer
5√7 / 2
PQRS is an isosceles trapezium in which PQ is parallel to RS and X is the mid point of PS. If PX= 8 and angle QXR= 90 degrees. Fine the perimeter of the trapezium PQRS.
Correct Answer
48
Length of sides of two rhombuses differ by 2. One of the diagonals for each of the rhombuses is of the same length as that of its side. Product of their areas is 168.75. Find the side of smaller
Correct Answer
3
BD and CE are the medians of the triangle ABC right angled at A. If CE = 5√13/2 and BC = 10, then the length of BD is:
Correct Answer
5√7 / 2
Correct Answer
√37 + √13
Correct Answer
1000
Correct Answer
7
Correct Answer
3π + 10
Correct Answer
2

We at Quantifiers understand and deliver on the personal attention each of our students requires. Whether it is through our pedagogy that enables non-engineers or non-math background students, our constant effort to proactively provide solutions, or our focus on our student’s goals.