Contact Us

DILR Condition Based
Set 2

Practice Questions for CAT with Solutions

1. CAT - DILR Condition Based Set 2

Priya wants to have a sandwich for dinner. She decides to go to the mall from home and buy all the required ingredients. It takes her 15 minutes to travel from home to the mall and vice versa. The following table represents all the required ingredients and stores (within the mall) from which they can be bought:

Priya takes 15 minutes to select any ingredient. Once the items are selected, the time required to go from one store to another and to get the billing done is negligible. Priya reaches home at 8:30 PM, after buying all the ingredients and traveling back and forth in the minimum possible time. Also,

  • Priya bought cheese just before the relevant store closed.
  • Mint sauce was not the last product selected at Naik Kirana Store.
  • Jalapenos were not selected immediately before Bread or Chilli Sauce.
  • Priya kept the gap between selecting tomatoes and tomato ketchup minimum.
  • Priya visited each store only once.

DILR Condition Based Set 2

Q1. At what time does Priya buy Mint Sauce?   

  1. 6:15 – 6:30 PM
  2. 7:00 – 7:15 PM
  3. 8:00 – 8:15 PM
  4. None

Q2. Which was the fifth ingredient to be selected?

  1. Tomato Ketchup
  2. Chili Sauce
  3. Jalapenos
  4. Mint Sauce

Q3. What was the time interval (in hours) between the time Priya finished selecting Lettuce and she started buying Chilli Sauce?

  1. 1
  2. 1.25
  3. 0.75
  4. 0.5

Q4. Priya decided not to adhere to her condition that Tomatoes and Tomato Ketchup had to be purchased within the minimum possible gap. In how many ways could she have completed all her purchases?

Correct Answer 1

d. None

Correct Answer 2

c. Jalapenos

Correct Answer 3

b. 1.25

Correct Answer 4

16
Video Explanation

2. CAT - DILR Condition Based Set 2

There are seven students (A, B, C, D, E, F, G) who have decide to read one book out of B1 – B7 and one novel out of N1 – N7 on each day of the week from Monday to Sunday. Exactly one book and one novel is read every day. They read them in two slots- Morning and Evening – on each day. No friend reads the same book or novel twice and only one friend reads on a given day.

  1. 3 novels were not read in the second slot and exactly two of them were of the same type (odd/even).
  2. E reads an odd numbered novel and book on Thursday in the first and second slot respectively.
  3. C and F did not read novels in the first slot during the weekend i.e. on Saturday or Sunday.
  4. G read B5 in the first slot and N1 in the second slot on Friday.
  5. A read the same numbered book and novel.
  6. B2, N5 and N2 were read on alternate days in the same order in the first slot.
  7. B2, B4 and B3 were read on consecutive days but not necessarily in the same slot nor order.
  8. On Saturday the difference between the book number and novel number was 5 between the two slots.
  9. The first novel of the first slot was read on Wednesday and A read N6 on Sunday in the second slot.
  10. B1 was not read on Monday and D read the highest numbered novel in the first slot

Q1. How many distinct arrangements are possible, considering the people, the books and novels that they read and the days on which they read them?

  1. 1
  2. 2
  3. 8
  4. CBD

Q2. Who reads N2 and on which day? 

  1. B, Wed
  2. B, Sat
  3. D, Wed
  4. D, Sat

Q3. If F reads on Monday, then who reads N4?

  1. F
  2. D
  3. C
  4. CBD

Q4. How many books and novels have been read before B1?

  1. 7
  2. 5
  3. 6
  4. CBD

Correct Answer 1

c. 8

Correct Answer 2

b. B, Sat

Correct Answer 3

d. CBD

Correct Answer 4

a. 7
Video Explanation

3. CAT - DILR Condition Based Set 2

There are five parties – A, B, C, D and E – contesting in an election. There is a bet on the probable winner. As per the betting rules, if a person bets on a party and if

  1. The party wins the elections; the person gets five times the amount bet.
  2. The party comes second in the elections; the person gets two times the amount bet.
  3. The party comes third in the elections; the person gets back the exact amount bet.
  4. If the party comes fourth or fifth, the person forfeits the amount bet.

A person can bet on a maximum of three parties. In the election results, it is known that C came second

Q1. Ashok bets Rs.5,000 on A, Rs. 3,000 on B and Rs. 1,000 on D. In how many ways can A just recover his amount?

Q2. Saumya bets Rs. 8,000, Rs. 5,000 and Rs. 2,000 on parties B, C and D (in no specific order) and gets a 20% return. What is the best possible rank for party E?
Q3. Varsha bets Rs. 500 each on three parties and gets a return of atleast 100%. In how many ways can she bet? Ignore the condition that C is second.
Q4. Varsha bet on parties B, C and D but lost money. What is the least numerical value of the sum of ranks of parties A and D?  Consider data from the previous question.

Correct Answer 1

0 ways 

Correct Answer 2

1st Rank 

Correct Answer 3

1080

Correct Answer 4

3
Video Explanation

4. CAT - DILR Condition Based Set 2

Akshay, Manoj, Pradeep, Vishal, Vinod and Priya received a total of fifty presents for Christmas. The difference in the number of presents received by Manoj and Akshay is same as that received by Vinod and Priya.
  • Tina’s office is one floor above Sharma’s and two floors above the Reliable Liquid Fund manager’s.
  • Mr. Jain isn’t the Reliable Debt Fund manager.
  • Kiran and Verma worked for the same mutual fund company before joining Reliable.
  • Priyal’s office is one floor above the Reliable Blue Chip Fund office.
  • The Reliable Worldwide Fund office is on the floor directly below where Singh has an office.
  • Karan, who isn’t Jain, managed the Liquid Fund before moving on last year to the fund he currently manages.
  • Verma’s office is one floor below that of the Debt Fund manager.
  • Mr. Sharma doesn’t manage the Reliable Sectoral Fund.
  • Tahil’s fund isn’t the Reliable Equity.
  • The Reliable Sectoral Fund manager has an office one floor above Julie, who is on the floor directly above fellow manager Jain.
  • Chopra’s office isn’t the one on the third floor of the Reliable Building

Q1. Who among the following manages the Equity fund?

  1. Tina
  2. Priyal
  3. Kashyap
  4. Kiran

Q2. Which of the following pairs has the maximum difference in the number of floors in between them?

  1. Kashyap – Singh
  2. Tina – Chopra
  3. Kiran – Chopra
  4. Kashyap – Tahil

Q3. The Sectoral Fund has its office on which floor of the building

  1. 5
  2. 6
  3. 4
  4. 3

Q4. What is the square of the sum of the floor numbers of offices of Kiran, Tina and Priyal?

  1. 36
  2. 64
  3. 81
  4. 100

Correct Answer 1

a. Tina

Correct Answer 2

c. Kiran - Chopra

Correct Answer 3

d. 3

Correct Answer 4

d. 100
Video Explanation

5. CAT - DILR Condition Based Set 2

Four friends A, B, C and D played a game of Poker. Initially, the number of chips that they had was in the 60s, 40s, 50s and 70s respectively, such that each one of them had a prime number of chips. They played 6 rounds of game such that the chips were only transferred among them. It is also known that:

  1. A lost 10 chips in each round and still finally ended up with a prime number of chips.
  2. The number of chips with B in each round formed an increasing arithmetic progression.
  3. The chips with D were all the possible numbers between 71 and 77 (both inclusive), but not in any specific order. No number in this range was excluded.
  4. B ended up with the number of chips that A had at the start of the game.
  5. The number of chips with D were even after rounds 1, 3 and 5 while the numbers were odd after rounds 2, 4 and 6.
  6. The number of chips with C after rounds 1 and 4 was a perfect square .
  7. Both C and D had 74 chips at the end of round 3.
  8. Everyone except D ended up with a prime number of chips.
Q1. What was the total number of chips used in the game?
Q2. At the end of how many rounds did C have more chips than everyone else?
Q3. After which round was the difference in the number of chips with A and B the least?
Q4. At the end of which round did D have 75 chips?

Correct Answer 1

240

Correct Answer 2

3 Rounds

Correct Answer 3

Round 2

Correct Answer 4

Round 2
Video Explanation

6. CAT - DILR Condition Based Set 2

Four friends: Nonie, Sophie, Rosie and Princie were to go to hill stations – Darjeeling, Shimla, Kodaikanal, Mussoorie – not necessarily in that order. Only one location was to be visited by one person and no person was to visit more than one location.
  1. Nonie will not go to Shimla unless Sophie goes to Darjeeling and Rosie goes to Kodaikanal.
  2. Sophie will not go to Kodaikanal unless Princie goes to Mussoorie and Nonie goes to Darjeeling.
  3. Rosie will not go to Mussoorie unless Nonie goes to Shimla and Sophie goes to Darjeeling.
  4. Nonie will not go to Darjeeling unless Princie goes to Mussoorie and Rosie goes to Kodaikanal.
  5. Sophie will not go to Darjeeling unless Nonie goes to Mussoorie and Rosie goes to Kodaikanal.
  6. Nonie will not go to Mussoorie unless Sophie goes to Shimla and Princie goes to Darjeeling.
  7. Princie will not go to Mussoorie unless Sophie goes to Shimla and Nonie goes to Kodaikanal.
  8. Nonie will not go to Kodaikanal unless Sophie goes to Mussoorie and Rosie goes to Shimla.
  9. Princie will not go to Darjeeling unless Rosie goes to Shimla
Q1. Who would have gone to Shimla?
  1. Nonie
  2. Sophie
  3. Rosie
  4. Princie
Q2. Who among the following could visit Darjeeling?
  1. Nonie
  2. Sophie
  3. Rosie
  4. Princie

Q3. Sophie could visit which of the following locations?

  1. Shimla
  2. Darjeeling
  3. Mussoorie
  4. Kodaikanal
Q4. If Nonie visits the place which Sophie was scheduled to go and Sophie visits the place which Rosie was to go, then which place could Rosie visit?
  1. Shimla
  2. Darjeeling
  3. Mussoorie
  4. No possible combination

Correct Answer 1

c. Rosie

Correct Answer 2

d. Princie

Correct Answer 3

c. Mussoorie

Correct Answer 4

d. No possible combination
Video Explanation

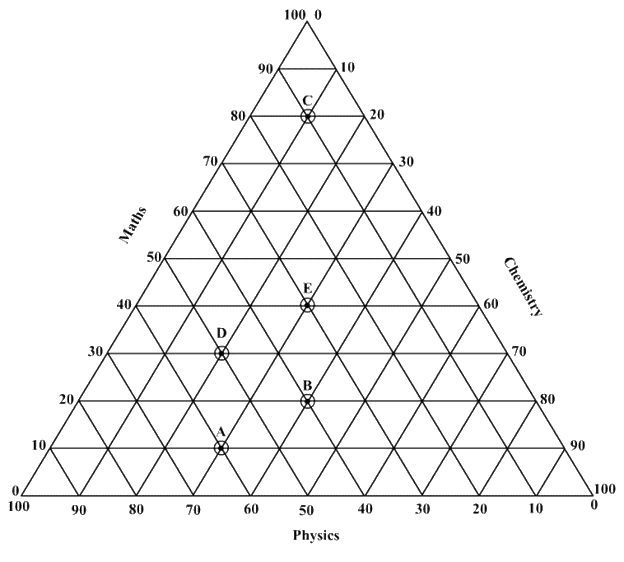
7. CAT - DILR Condition Based Set 2

Eight friends – A, B, C, D, E, F, G, H – have among them apple, banana, orange, guava, papaya, mango, pomegranate and carrot, in that order. The total number of fruits between them is 36 and no two friends have the same number of fruits. Each person has exactly one type of fruit and at least one unit of that fruit.
Further, it is known that:
  1. The total number of apples and papayas is the same as number of pomegranates that G has.
  2. There are ten oranges and guavas put together.
  3. The total number of papaya and mangoes is eight.
  4. There a half a dozen apples and oranges in all.
  5. D has the maximum pieces of fruits.
  6. The number of fruits with E is not a perfect square.
Q1. How many papayas does E have?
Q2. The number of fruits with G is?
Q3. How many mangoes were there?
Q4. What was the total number of carrots and bananas?

Correct Answer 1

3

Correct Answer 2

7

Correct Answer 3

9

Correct Answer 4

7
Video Explanation

8. CAT - DILR Condition Based Set 2

The percentage of marks of five students A, B, C, D and E in three subjects Physics, Chemistry and Maths are represented in the triangular graph above. For a subject, percentage is taken by first moving along that axis from the vertex marked 0 for that subject and stopping at the point where the student’s percentage in that subject first crosses that particular axis. The maximum marks in each subject are 200.

DILR Condition Based Set 2

Q1. What are the average marks obtained by these students in Chemistry?
Q2. P is another student whose marks are marked on one of the inner vertices of the same graph. His average marks per subject are between:
Q3. F is another student whose marks are plotted on the same graph. Marks obtained by A and F in Maths are in the ratio 2 : 5 and marks obtained by C and F in Physics are in the ratio 2 : 7. What is the percentage of marks obtained by F in Chemistry?
Q4. X is the only topper in Maths and lies on one of the inner vertices. What is the least difference in his and D’s marks in Chemistry

Correct Answer 1

52

Correct Answer 2

66.66

Correct Answer 3

40%

Correct Answer 4

20
Video Explanation

9. CAT - DILR Condition Based Set 2

A train starts at station 1 and goes to station 8 via six stations – 2 to 7 – in that order. At each of these six stations, twice as many people get in as those that get down. The number of people getting down at these six stations are all prime numbers – one each between 0 and 10, 10 and 20, and so on between 50 and 60, in the order of the stations given above. In these six stations, the difference between the number of people getting down at any two consecutive stations is at least ten. The number of people who got down at station 7 is more than 55. The total number of passengers getting down at all six stations together is an even number
Q1. How many people got down at station [6]?
Q2. The train started with 32 people. All the passengers left in the train at station 8 got down there. How many people got out of the train at station 8?
Q3. Which of the following cannot be the number of people getting down at any station?
  1. 47
  2. 3
  3. 17
  4. 23
Q4. What is the minimum number of people that the train must have started with at station 1?
  1. 10
  2. 11
  3. 3
  4. 8

Correct Answer 1

47

Correct Answer 2

214

Correct Answer 3

c. 17

Correct Answer 4

a. 10
Video Explanation

10. CAT - DILR Condition Based Set 2

7 friends P, Q, R, S, T, U, V parks their cars in a straight line, one behind the other. U’s car is always behind V’s. Q’s car touches P’s & T’s. R parks his car just behind T’s. S’s car and P’s car cannot be in the neighbouring positions. Consider that all the cars face the west.
Q1. The cars can only leave from the western gate of the society, which opens at 3:15 p.m. The first car leaves n minutes after the gate has opened and each subsequent car leaves 2n minutes after its preceding car, where n corresponds to the alphabetical position of the name of the car that is about to leave. In how many ways can the cars be arranged if T does not want to leave before 4:40 p.m on the same day?
  1. 1
  2. 3
  3. 7
  4. 9
Q2. Which car, if identified as the one parked behind all the others, would give the maximum number of arrangements?
  1. U’s Car
  2. V’s Car
  3. S’s Car
  4. S’s Car
Q3. Which data about a car and its position will not give a unique arrangement? (Consider that the last car is the car that is parked behind all the others i.e., east-most as compared to remaining six cars.)
  1. S’s car is in the second position
  2. P’s car is in the third position
  3. V’s car is the second last car
  4. None of these
Q4. The cars are parked in such a way that only the extreme end cars can move without any other car moving. In any possible arrangement, what is the difference between the cars that need to move for P to move his car and cars that need to move for T to move his car?
  1. 1 or 3
  2. 2
  3. 0 or 3
  4. 0 or 2

Correct Answer 1

d. 9

Correct Answer 2

a. U’s Car

Correct Answer 3

b. P’s car is in the third position

Correct Answer 4

d. 0 or 2
Video Explanation

Newsletter Subscription

Subscribe to our newsletter and stay updated with all the latest news, tips, and information about the CAT exam delivered directly to your inbox.

Newsletter Subscription

We at Quantifiers understand and deliver on the personal attention each of our students requires. Whether it is through our pedagogy that enables non-engineers or non-math background students, our constant effort to proactively provide solutions, or our focus on our student’s goals.

Useful Links

Video Resources

Free Tests

Contact Us

© Copyright Quantifiers. All Rights Reserved.

Privacy Policy